On The Effects Of Ballpark Altitude On The Number Of Home Runs

The other day a friend of mine commented that he witnessed quite a few home runs during a Cubs vs. Rockies game at Coors Field in Denver. There was a total of 4 home runs, including back-to-back solo home runs by the Cubs early in the game. I immediately posited that it was likely due to the higher altitude of the field (5,197 ft.) versus the rest of the stadiums in the Major Leagues. A combination of dry western air along with the lower density at altitude, should be ideal for allowing balls to travel longer distances. In fact, after the stadium opened for the 1995 season, I remember hearing much in the news about the number of home runs, triples and doubles that occurred in the stadium. I was mid-way through my graduate thesis at the time and didn’t pay much attention to the sport back then. Later during the week, it dawned on me that I hadn’t heard as much about the effect in recent times. I wondered if there really was an altitude effect at work. It turns out that a basic Google search could have answered my suspicion, but I will not ruin some good data analysis for you just yet.

There is a discussion about the effects of high altitudes in The Physics of Baseball, a fantastic book by Robert K. Adair that is now in its third edition. Before I jump right in to the data analysis, let’s first recap the physics involved in the flight of a baseball.

Once a ball is launched in flight there are only two forces acting upon it. First, there is gravity working to bring the ball back to the ground and second, friction due to air resistance which works to slow the ball down or causes it to change direction. This friction has two components. One of these is normal air resistance (drag), the collision of the ball with the millions of air molecules in its flight path, that works against the ball’s direction of motion and cuts short the overall distance it will travel. The other component is called the magnus effect, a deflection of a spinning ball based on the spin imparted by a pitcher (in the case of a pitched ball) or imparted by the bat based on where it strikes the ball. Essentially, a spinning ball traveling through the air will tend to curve in the direction where the spin is aligned with the air moving over the ball’s surface. Consider the classic pitcher’s sinker. If a pitcher can release the ball while putting a forward spin, also called top spin, on the ball then the bottom side of the ball will be moving in the same direction as the air flowing passed it (smooth airflow, less resistance). The top side of the ball will be moving against the airflow (more air resistance and turbulence). The difference in pressure created on the two sides of the ball causes it to deflect downwards. This is why fastballs tend to sink much faster than an ordinary tossed ball with little or no spin. Other pitches will deflect in other directions depending on the type of spin a pitcher imparts on the ball.

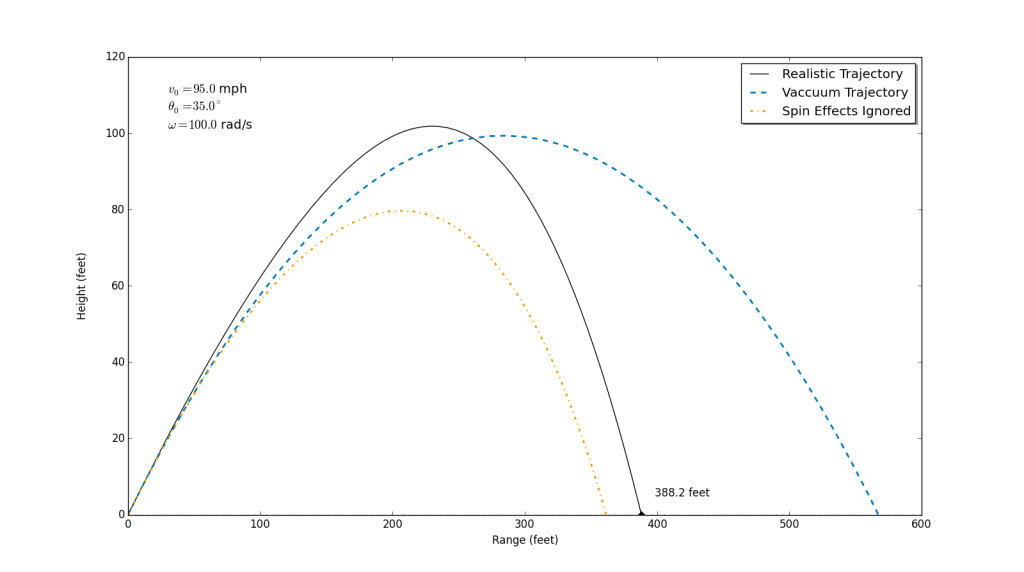
Without air, we expect that a ball coming off of a bat will follow a perfect arc, a parabolic trajectory, from start to finish. Air resistance works to cut short the ball’s path so what we have is a trajectory that starts off parabolic but then shows a sharper drop to the ground towards the end of the flight. A batted ball spins as well. In fact, most home runs occur when the ball contacts the part of the bat just above the medial axis, giving the ball back-spin. The magnus effect then tends to deflect the ball upwards and keeps it in flight longer than it otherwise would. The first plot below is a simulation that shows three different cases where a ball is batted with the same initial angle and starting velocities. The blue dashed line shows how the ball would travel if there was no air resistance. The dotted tan line shows the trajectory the ball would take when we include air resistance but ignore spin effects (magnus effect). The solid black line shows the actual trajectory the ball takes.

Baseball Trajectories (D.O. Gunter, Python calculations)

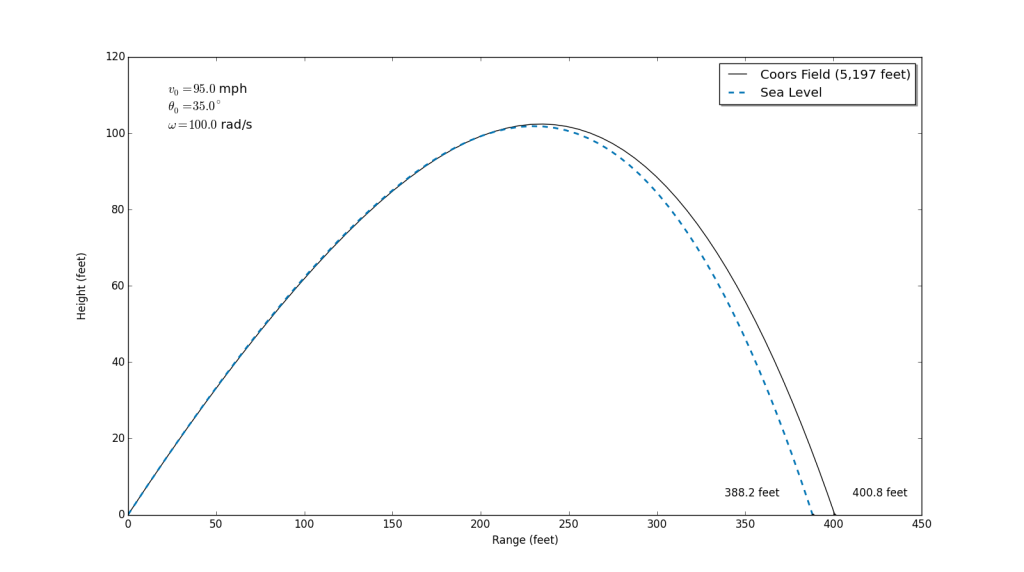
Now that we understand how the various forces act upon a baseball in flight let us see how a change in altitude can affect things. The next plot shows the actual trajectories of a batted ball with the same initial parameters as before but for two different starting altitudes, Coors Field in Denver at 5,197 feet and another at sea level. The ball batted at high altitude travels just a bit further. Using typical values of batted balls, we see that it is just far enough to push it over the outfield walls.

Balls batted at higher altitude travel further (D.O. Gunter, Python calculations)

An increase in altitude means a decrease in the ambient air density. The air density in a high altitude stadium such as Coors Field is about 80% of that in a stadium at sea level, all other things being equal (temperature, wind speed, etc.). That means there will be an 80% decrease in the effect of air resistance on the ball and an 80% decrease in the magnus effect on the spinning ball. The magnus effect is much weaker than the drag due to air resistance, so a ball hit under those conditions should travel further than it would at sea level. This should mean that more home runs would be hit in such a stadium. As a side note, these same issues affect the pitching of the ball to the batter’s advantage. A breaking ball that curves upwards as much as 8-inches by the time it reaches the plate will only move about 6-inches in a stadium at high altitude. This makes it more difficult to fool the batter and leads to more opportunities for him to hit the ball.

Now that we have the theoretical stuff out of the way, let us find out what the data has to tell us. The fantastic team over at HitTrackerOnline.com have been keeping tabs on MLB home runs for the last several years. Their database is a science nerd’s dream cache. They record every home run during every game. They record such information as the time, place, temperature, wind speed and direction, the ball speed off the bat, the vertical and horizontal angles it was launched from, and the apex of its trajectory. We can even see the trajectory overlaid on a diagram of the stadium for each and every home run. It is beyond impressive.

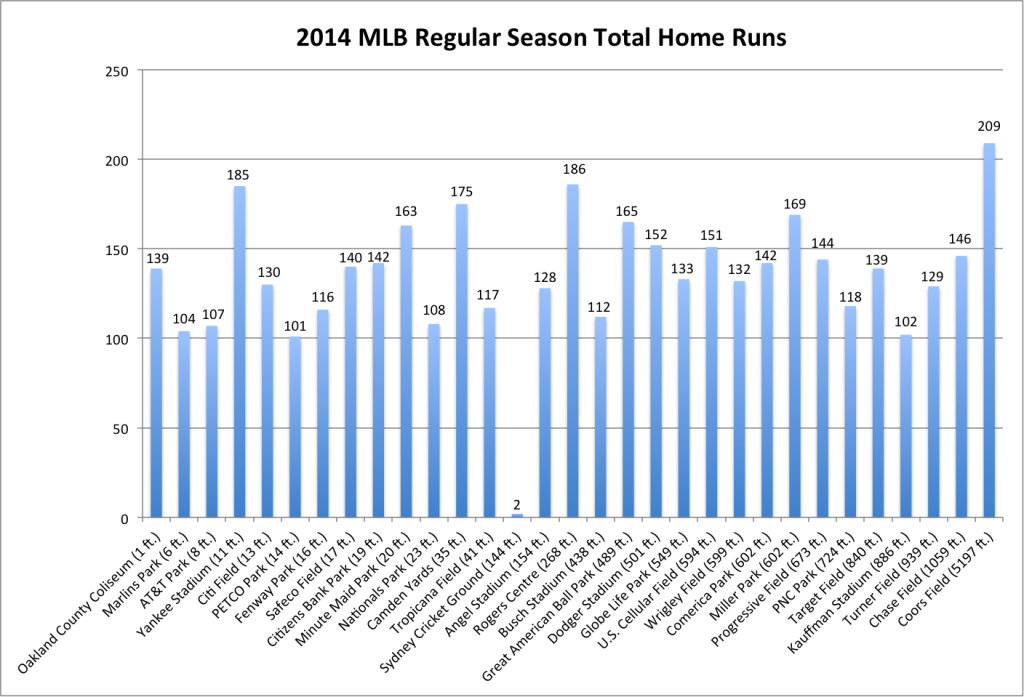
I have collected the 2014 regular season home runs into a spreadsheet to help analyze the effects of the altitude of the stadium. The first chart below shows the total number of home runs per stadium. The stadiums are arranged from lowest to highest altitude from left to right. As expected, there were quite a few more homers hit in Coors Field. Also notice the dip in the middle. The 2014 MLB season began with two games in Australia’s Sydney Cricket Grounds. Also note that there were a large number of home runs hit in Yankees Stadium and in Rogers Centre. The Yankees and the Blue Jays have some heavy hitters on the payroll, so it isn’t quite clear that the high mark of Coors Field is strictly due to altitude or simply due to having better hitters on their roster as well. (I should find a roster of all players and look at the hitting performance. Look for a future post.)

The total number of home runs per stadium during the 2014 MLB regular season.

There is another important aspect that will affect the number of home runs hit in a particular stadium. The position of the outfield walls is not something that is standard across stadiums in the league. There are some stadiums such as Petco Field (pre-2012 modifications) in San Diego where no home run will occur unless the ball travels at least 400 feet or more. Then there are those stadiums such as Cincinnati’s Great America Ball Park and San Francisco’s AT&T Park where a significant portion of the left and right outfields end at 350 feet. Coors Field is a reasonably new stadium with typical outfield wall distances around the 380 to 400 foot distances. In fact, if we overlay the diagrams for Yankees Stadium or Rogers Centre on top of the Coors diagram, you see that those two stadiums are quite a bit shallower than Coors Field. The case for the altitude effect is starting to look good.

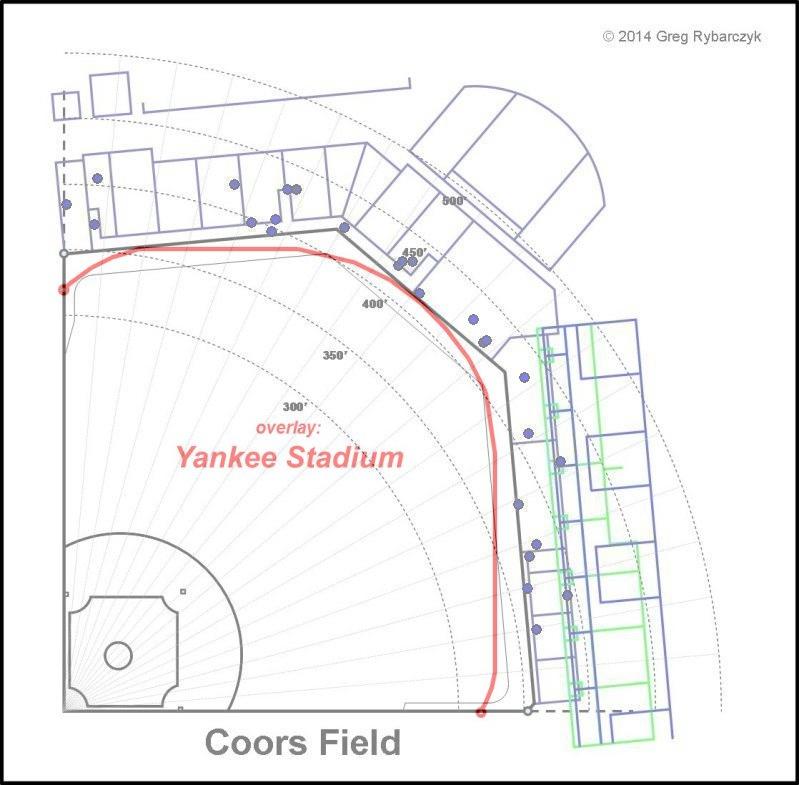
Coors Field layout with overlay showing field size of Yankees stadium (http://www.hittrackeronline.com/)

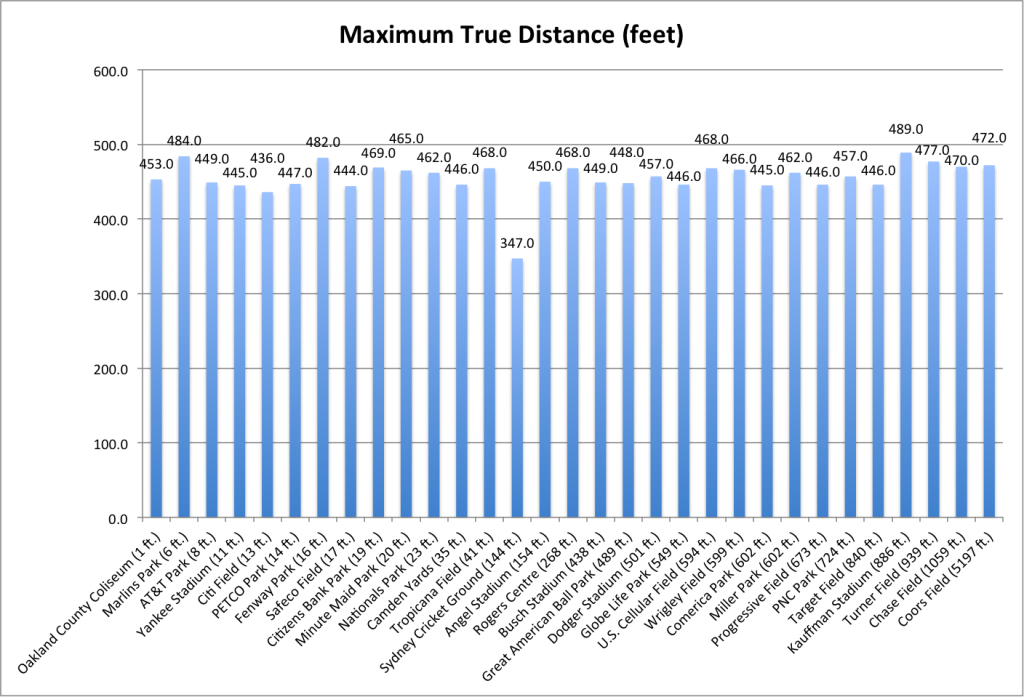
The number of home runs hit per stadium does not provide enough evidence to completely support the altitude effect so next we will examine the true distance traveled by each home run ball. Now, what is the true distance? It is defined as the total horizontal distance that each ball would travel if it were allowed to return to the ground. It is a calculated number but one based on the fairly accurate data gathered at the time of each hit. The reason this distance is important is because many home run balls do not travel very far once they pass over the outfield wall. They may get caught by fans, fall into a fountain, or bounce off the scoreboard, and so on. By knowing how fast the ball left the bat, the angles with which it was launched, the apex, the temperature, the wind speed and direction, and other information, it is very easy to compute the actual trajectory the ball would have travelled with no obstacles in the way. This calculation was done by the crew at the HitTracker website and is already part of the dataset. The next chart is a plot of the maximum true distance among all the home runs for each stadium, again arranged from lowest to highest altitude stadiums. There are 4 hits that were longer than the longest home run ball from Coors Field. This doesn’t help make the case that Coors Field has an altitude advantage. The distribution of lengths across the various altitudes looks more like a random distribution.

The maximum home run distance from each stadium.

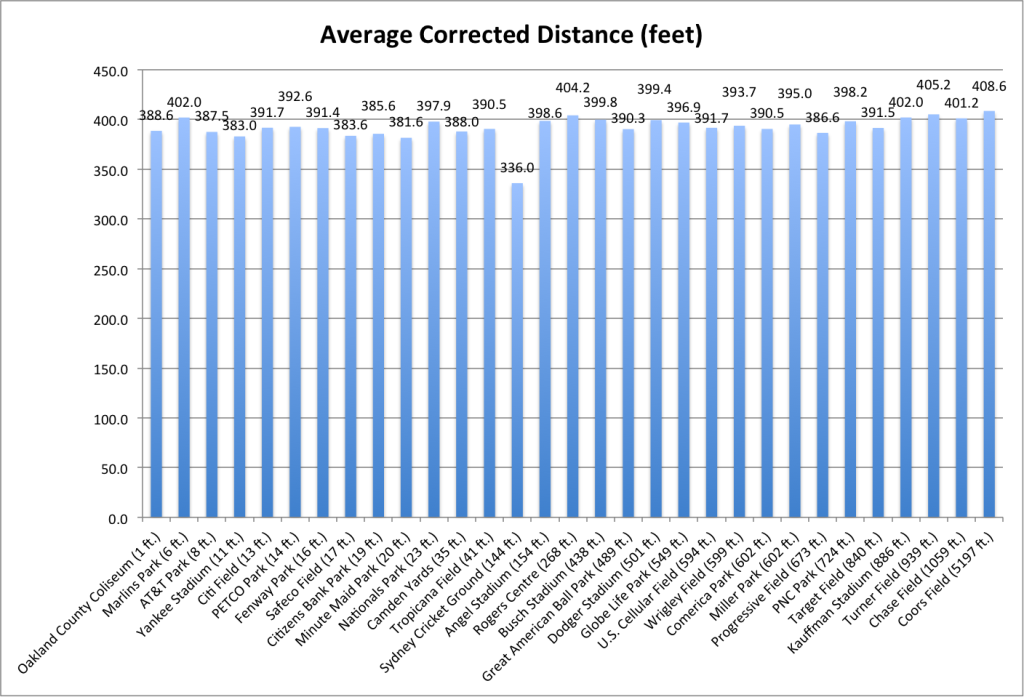
This makes sense because we are only looking at the best home run from each stadium and this has a lot to do with the power of the hitter, the “luck” of making a perfect contact, etc. It would be more statistically significant to look at the average of all the true distances and this is what is shown in the next chart. Here we definitely see that Coors Field comes out on top even if only slightly so.

The average true distance per stadium.

One fact we have ignored until now is that all these home runs were hit in varying temperature and wind conditions. If we truly want to isolate the effects of altitude alone on a ball’s distance (and eventual home run status) then we need to correct the true distance of each of these home run flights using the other information recorded at the time. Correction factors have been calculated to give the true distance the balls would have taken if they were hit in 75°F temperature, no-wind conditions, at their respective altitudes. The average of these corrected true-distances is presented in this last chart.

The average of the true distances corrected to standard weather conditions.

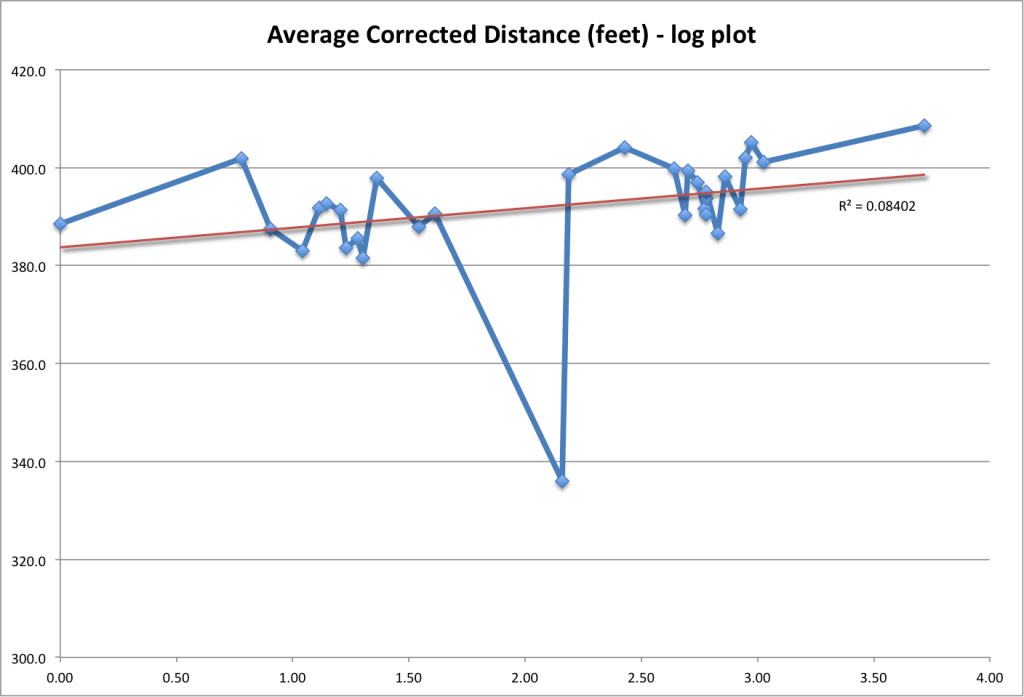
This, too, shows only a slight advantage to hitting a ball in Coors Field. It is instructive at this point to plot the actual average true corrected distance as a function of altitude. Up to now we have only seen charts with equally spaced bars along the horizontal axis. Of course, with Coors Field sitting so much higher than the other 30 stadiums in the list, such a graph would have most of the data points skewed to the left edge. Instead, we plot the log of the stadium altitude versus the average true corrected distance.

A log plot of the average true corrected distance versus the log of the stadium altitudes.

This plot shows some interesting features. The left third of the plot shows the cluster of 13 stadiums that sit at less than 50 feet above sea level. The home run distances tend to hover around an average distance that is noticeably less than that of the next cluster of stadiums which are the inland stadiums sitting between 200 and 900 feet above sea level. Finally, Coors Field seems to be off on its own, at a much higher 5,197 feet of elevation. The trend line that represents a best fit of the data indicates that we would expect a ball to travel 18 more feet in Coors Field than if hit at sea level in Oakland County Coliseum. The R-squared value of that fit does nothing to instill any confidence in this, however.

I think it is safe to say that yes, there is some overall advantage to hitting a ball in a high altitude stadium like Coors Field. My Python simulations accurately model the atmosphere, the dynamic drag and spin coefficients of real baseballs, and they clearly show a pronounced effect. So why isn’t this seen in the actual data? There should be even more home runs at Coors Field than are actually hit, so something is awry. Either the data is wrong or my physics modeling is far from ideal.

It turns out that neither of those two possibilities is at play. To see this, look at the data up until 2002 (not available at HomerunTracker.com). From the day it opened until the 2002 season Coors Field was a hitters dream, a virtual home run factory. The number of home runs hit in that park far exceeded all the others without having to rely on sophisticated model corrections. (I did not come to learn of this until after gathering the 2014 data set and doing my analysis.)

What changed? Before the start of the 2002 season, MLB officials and the Rockies organization agreed to build a room-sized humidor in which to store balls used for games. The balls are kept moist so that they end up being slightly heavier than regular game balls and thus, do not travel nearly as far with the same impact. This had the effect of leveling the playing field, as they say. You can read about this in this Denver Post article. It is a shame I do not have access to any of the data for those early years, but a study of the humidor effect showed that the number of home runs hit in the stadium decreased by as much as 25%. That is rather significant.